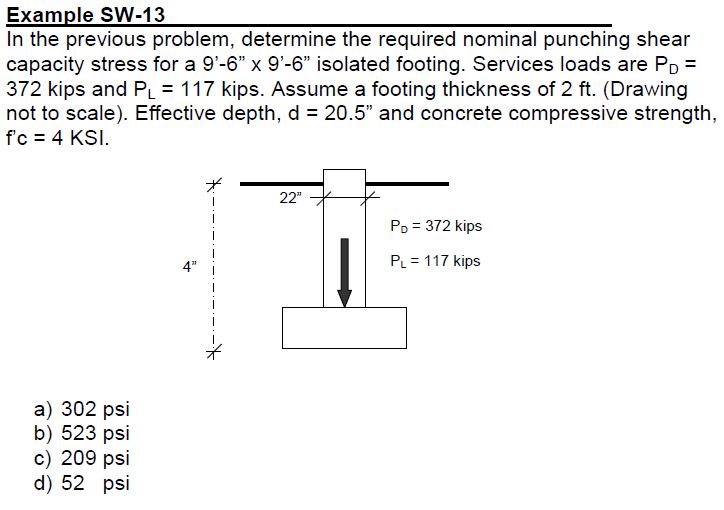
Shear Capacity Stress Question

[CE_Gator]

 

[civilized_naah]

Going by memory (of formulas etc.) because I don't have any books here, I am not getting any of the given choices. I am getting the punching shear stress under factored (Ch. 9) loads = 156 psi and the design punching shear stress limit of 190 psi

 

[ptatohed]

This topic is way beyond my level but simply using an example out of my old CERM10 (outdated?), and plugging+chugging, this is what I get:

b1 = b2 = 12" + (2) (d/2) = 12" + (2)(20.5") = 32.5"

Ap = 2(b1 + b2)d = 2(32.5" + 32.5")(20.5") = 2665.0in2

Pu = (1.4)(Pd) + (1.7)(Pl) = (1.4)(372k) + (1.7)(117k) = 719.7k

R = Pu((b1 x b2))/Af = (719.7k)(32.5")(32.5")/(114in2) = 58.49k

vu = (Pu - R) / Ap = (719.7k - 58.49k) / 2665.0 in2 = 0.2481 kips/in2 = 248.1 lb/in2

Just Like c_n, I don't get one of the available answers.

Are my calcs bad?

 

[Porter_]

Using CERM 13th edition I get the same as civilized_naah:

b1 = b2 = 42.5"

Ap = 2(42.5" + 42.5")(20.5") = 3485 in2

Af = 114" x 114" = 12996 in2

Pu = (1.2)372k + (1.6)117k = 633.6k

R = (633600 lbs)(42.5")(42.5")/12996 in2 = 88061 lbs

Vu = (633600 lbs - 88061 lbs)/3485 in2 = 156 psi

The nominal concrete shear stress is:

Vc = (2+2)(.75)(4000)^(1/2) = 190 psi (assuming lightweight concrete)

 

[civilized_naah]

To get the 190 psi, I calculated the DESIGN (rather than NOMINAL) shear stress as phi v_c = 0.75 v_c. I did not assume lightweight concrete (typically use normal weight concrete for footings)

 

[Porter_]

Ah I see. Is that from ACI 318 section 9.3.2.3? (I'm green here; in the process of learning).

 

[ptatohed]

Using CERM 13th edition I get the same as civilized_naah:

b1 = b2 = 42.5"

Ap = 2(42.5" + 42.5")(20.5") = 3485 in2

Af = 114" x 114" = 12996 in2

Pu = (1.2)372k + (1.6)117k = 633.6k

R = (633600 lbs)(42.5")(42.5")/12996 in2 = 88061 lbs

Vu = (633600 lbs - 88061 lbs)/3485 in2 = 156 psi

The nominal concrete shear stress is:

Vc = (2+2)(.75)(4000)^(1/2) = 190 psi (assuming lightweight concrete)

Thanks Porter. It looks like my CERM10 is outdated (with respect to ACI 318).

What is the new formula for b1, b2? I guess it is not 12in + 2(d/2) anymore?

Also, it looks like Pu changed from 1.4Pd + 1.7Pl to 1.2Pd + 1.6Pl, correct?

Thanks.

 

[civilized_naah]

What is the new formula for b1, b2? I guess it is not 12in + 2(d/2) anymore?

Also, it looks like Pu changed from 1.4Pd + 1.7Pl to 1.2Pd + 1.6Pl, correct?

Thanks.

The critical section for punching shear is located d/2 from the face of the COLUMN - so the dimensions are calculated by adding d to the COLUMN DIMENSION which is 22 inches, not 12 inches in this example .

Also, the load combination in Chapter 9 is 1.2D+1.6L. The 1.4D+1.7L is relegated to Appendix C (which the PE syllabus says will NOT be used in formulating answers

 

[Porter_]

Using CERM 13th edition I get the same as civilized_naah:b1 = b2 = 42.5"Ap = 2(42.5" + 42.5")(20.5") = 3485 in2Af = 114" x 114" = 12996 in2Pu = (1.2)372k + (1.6)117k = 633.6kR = (633600 lbs)(42.5")(42.5")/12996 in2 = 88061 lbsVu = (633600 lbs - 88061 lbs)/3485 in2 = 156 psiThe nominal concrete shear stress is:Vc = (2+2)(.75)(4000)^(1/2) = 190 psi (assuming lightweight concrete)

Thanks Porter. It looks like my CERM10 is outdated (with respect to ACI 318).What is the new formula for b1, b2? I guess it is not 12in + 2(d/2) anymore?Also, it looks like Pu changed from 1.4Pd + 1.7Pl to 1.2Pd + 1.6Pl, correct?Thanks.

I think b1 and b2 are still the same formula, = (column width) + 2(d/2). You just used 12" instead of the 22" dimension given in the problem. Yeah it looks like your 10th edition is out of date, Pu = 1.2D + 1.6L per ACI 318-08 section 9.2.

 

[ptatohed]

Thanks guys. Boy am I glad I went with Transportation. :S

 

[BoilerEng]

Using CERM 13th edition I get the same as civilized_naah:

b1 = b2 = 42.5"

Ap = 2(42.5" + 42.5")(20.5") = 3485 in2

Af = 114" x 114" = 12996 in2

Pu = (1.2)372k + (1.6)117k = 633.6k

R = (633600 lbs)(42.5")(42.5")/12996 in2 = 88061 lbs

Vu = (633600 lbs - 88061 lbs)/3485 in2 = 156 psi

The nominal concrete shear stress is:

Vc = (2+2)(.75)(4000)^(1/2) = 190 psi (assuming lightweight concrete)

Bumping an old thread but wanted to make sure I'm looking at this correctly.

I agree with Porter's calcs, but wanted to add to it. There really does not seem to be a reason to calc Vc. The question is asking what the required stress should be, so them giving f'c just adds fluff to the problem.

To me, the required stress would be factored applied stress divided by phi = 156 psi / 0.75 = 208 psi, which is choice C. (209 psi).

Now, granted the footing does not meet this criteria, but the question is only asking what the required stress would be, not the actual two-way shear capacity is.