NCEES Power Sample Exam #530 and #519

[DK PE]

Hi Rei, take a look at the reference I listed at the other thread on prob #530.... maybe it will help. You can sort of think of it as trying to find the thevenin equivalent of the utility source upstream.

 

[knight1fox3]

Hi Rei, take a look at the reference I listed at the other thread on prob #530.... maybe it will help. You can sort of think of it as trying to find the thevenin equivalent of the utility source upstream.

I'm having a bit of trouble locating the thread you mentioned. Could you provide a link? Thanks.

 

[Flyer_PE]

^He's talking about this thread.

The paper he mentions is here.

 

[BamaBino]

The paper he mentions is here.

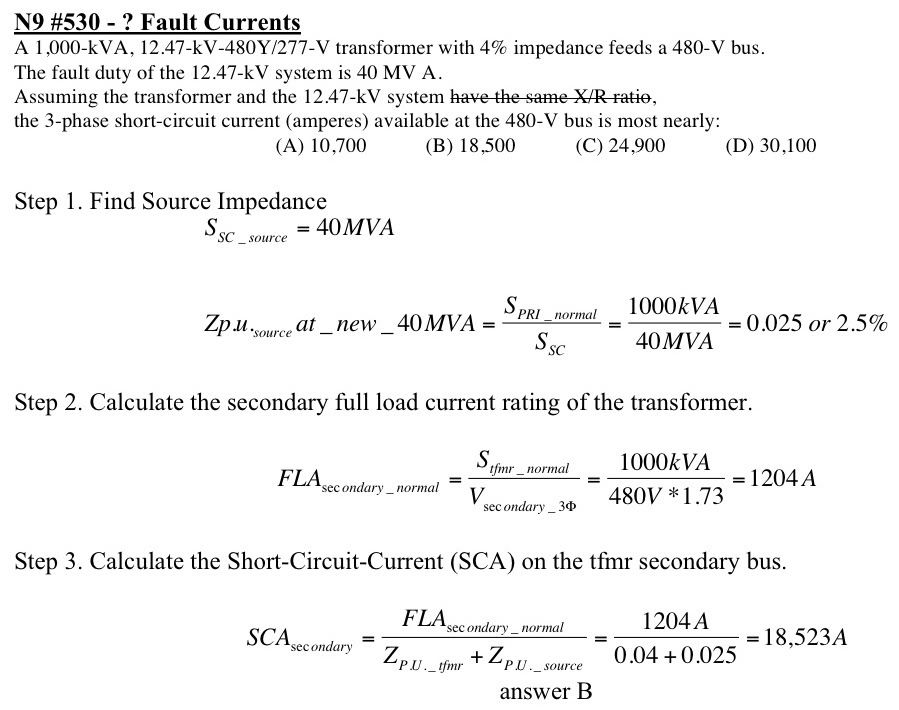
I worked NCEES # 530 using the same method as the example in that paper. I like the approach and it is the second jpeg below.

When tried working NCEES # 540 using the same general method, I had a problem getting the right answer (close but off 10%). I'm sure the error is in the last step or a step was left out. Would someone point out where it went wrong?

Thanks

 

[Flyer_PE]

I didn't do all the math. I do see one problem with your solution though. You can't add the pu impedance of the generator (834MVA base) to the pu impedance of the transformer (933MVA Base). It looks like you have chosen the transformer rating (933MVA) as the base for the system. You will need to convert the generator pu impedance from the 834MVA base to the 933MVA base of the transformer.

 

[BamaBino]

I didn't do all the math. I do see one problem with your solution though. You can't add the pu impedance of the generator (834MVA base) to the pu impedance of the transformer (933MVA Base). It looks like you have chosen the transformer rating (933MVA) as the base for the system. You will need to convert the generator pu impedance from the 834MVA base to the 933MVA base of the transformer.

That was the problem. Now I get the correct answer.

Thanks

 

[BamaBino]

I didn't do all the math. I do see one problem with your solution though. You can't add the pu impedance of the generator (834MVA base) to the pu impedance of the transformer (933MVA Base). It looks like you have chosen the transformer rating (933MVA) as the base for the system. You will need to convert the generator pu impedance from the 834MVA base to the 933MVA base of the transformer.

Below is Problem 540 after making this correction to get answer B.

 

[jayache80]

reading through this, I just wanted to add: by definition, S_sc = S_base / Zpu.

When they give us "fault duty" of 40MVA, that means they gave us S_sc, using 40MVA as S_base:

S_sc = S_base / Zpu

40MVA = 40MVA / Zpu

Zpu should be assumed to be 1pu, as Flyer has said, but it didn't click with me until I realized this definition.

As it's been said, I think the best way to solve these types of problems is using the MVA method:

Ssc_xfmr = S_xfmr_rated / Zpu = 1000kVA / .04 = 25MVA

Ssc_sys = 40MVA (given)

To find the total Ssc, when combining Ssc's in series, it's like resistors in parallel.

Ssc_total = Ssc_xfmr * Ssc_sys / (Ssc_xfmr + Ssc_sys)

Ssc_total = 25MVA * 40MVA / (25MVA + 40MVA)

Ssc_total = 15.3846 MVA (this number looks familiar if you did it with the per unit method first)

Now, to get your Isc, just 'three-phase' divide by the voltage of interest:

Isc = Ssc_total / (rad3 * V)

Isc = 15.3846MVA / (rad3 * 480) = 18,504.8 A

 

[saberger_vt]

Two things:

1. They really like giving you extraneous information in the problems. In that problem, the X/R ratios are totally irrelevant.

2. You can choose any base voltage you want. In this case it simplifies things if you choose 12.47 kV as the base because it makes the math real easy. Typically, the power and voltage bases are chosen based on either the system or the largest transformer in your analysis. In this problem, if you use something other than 12.47 kV, the 4% impedance value for the transformer will have to be adjusted to the new base and you will no longer be applying a 1 pu voltage to the fault. In the end, you'll still wind up with the same number of amps though.

I would agree with Flyer_PE that in this particular problem, the X/R ratio is irrelevant since it equals 1. From the point of solving short circuits, it is still a viable piece of information. Here is one post on engineersboard covering some calculations using the X/R, and another post of an older GE document covering asymmetrical faults:

X/R Ratio and 3 phase fault
GE Document:

Short-Circuit Current Calculations - GE Industrial Systems